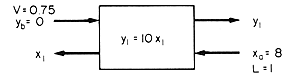Unit Operations in Food Processing

The separation which will be effected in a given series of contact stages can be calculated by combining the equilibrium and the operating relationships. Starting at one end of the process, the terminal separation can be calculated from the given set of conditions. Knowing, say, the x value in the first stage, x₁, the equilibrium condition gives the corresponding value of y in this stage, y_1. Then eqn. (9.5) or eqn. (9.6) can be used to obtain y₂ then the equilibrium conditions give the corresponding x₂, and so on ...

EXAMPLE 9.4. Single stage steam stripping, of taints from cream
A continuous deodorizing system, involving a single stage steam stripping operation, is under consideration for the removal of a taint from cream. If the taint component is present in the cream to the extent of 8 parts per million (ppm) and if steam is to be passed through the contact stage in the proportions of 0.75 kg steam to every 1 kg cream, calculate the concentration of the taint in the leaving cream. The equilibrium concentration distribution of the taint has been found experimentally to be in the ratio of 1 in the cream to 10 in the steam and it is assumed that equilibrium is reached in each stage.

Call the concentration of the taint in the cream x, and in the steam y, both as mass fractions,

From the condition that, at equilibrium, the concentration of the taint in the steam is 10 times that in the cream:

10x = y

and in particular, 10x₁ = y₁

Now, y₁ the concentration of taint in the steam leaving the stage is also the concentration in the output steam

y₁ = y_a = 10x₁

The incoming steam concentration = y₂ = 0 as there is no taint in the entering steam.

The taint concentration in the entering cream is x_a = 8 ppm.

These are shown diagrammatically in Fig. 9.3.
Basis is 1 kg of cream

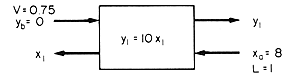
FIG. 9.3 Flows into and out from a stage
Figure 9.3 Flows into and out from a stage

The problem is to determine x₁ the concentration of taint in the product cream.

The mass ratio of stream flows is 1 of cream to 0.75 of steam and if no steam is condensed this ratio will be preserved through the stage.

1/0.75 = 1.33 is the ratio of cream flow rate to steam flow rate = L/V.

Applying eqn. (9.6) to the one stage n = 1,

                     y₂ = x₁L/V + y_a - x_aL/V
                     y₂ = 0 = x₁1.33 + 10x₁ - 8 x 1.33

                     x₁ = 10.64/11.33
                         = 0.94 ppm

which is the concentration of the taint in the leaving cream, having been reduced from 8 ppm.

This simple example could have been solved directly without using the formula, but it shows the way in which the formula and the equilibrium conditions can be applied.

Based on the step-by-step method of calculation, it was suggested by McCabe and Thiele (1925) that the operating and equilibrium relationships could very conveniently be combined in a single graph called a McCabe-Thiele plot

The essential feature of their method is that whereas the equilibrium line is plotted directly, x_n against y_n, the operating relationships are plotted as x_n against y_n+1. Inspection of eqn. (9.5) shows that it gives y_n+1 in terms of x_n and the graph of this is called the operating line. In the special case of eqn. (9.6), the operating line is a straight line whose slope is L/V and whose intercept on the y-axis is (y_a- x_aL/V).

Considering any stage in the process, it might be for example the first stage, we have the value of y from given or overall conditions. Proceeding at constant y to the equilibrium line we can then read off the corresponding value of x, which is x₁. From x₁ we proceed at constant x across to the operating line at which the intercept gives the value of y₂. Then the process can be repeated for y₂ to x₂, then to y₃, and so on. Drawing horizontal and vertical lines to show this, as in the Fig. 9.4, a step pattern is traced out on the graph. Each step represents a stage in the process at which contact is provided between the streams, and the equilibrium attained. Proceeding step-by-step, it is simple to insert sufficient steps to move to a required final concentration in one of the streams, and so to be able to count the number of stages of contact needed to obtain this required separation. [Fig. 9.4 both illustrates the general process, with two stages, and also gives numerical data to solve later Example (9.5) of a two-stage steam stripping/gas absorption process].

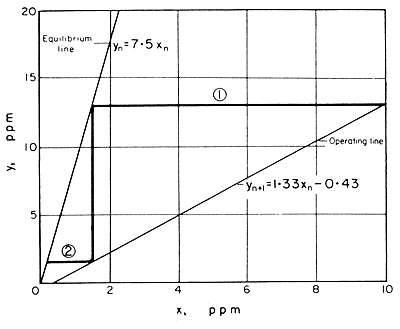
FIG. 9.4 Steam stripping: McCabe-Thiele plot
Figure 9.4 Steam stripping: McCabe-Thiele plot

On the graph of Fig. 9.4 are shown the operating line, plotting x_n against y_n+1, and the equilibrium line in which x_n, is plotted against y_n. Starting from one terminal condition on the operating line, the stage contact steps are drawn in until the desired other terminal concentrations are reached. Each of the numbered horoizontal lines represents one stage.This procedure is further explained in Example 9.5.

Contact-Equilibrium Processes - Part 2: APPLICATIONS > GAS ABSORPTION

Back to the top