To separate liquid streams, the liquids must be immiscible,
such as oil and water. Liquid-liquid extraction is the name used when
both streams in the extraction are liquid. Examples of extraction are
found in the edible oil industry in which oil is extracted from natural
products such as peanuts, soya beans, rapeseeds, sunflower seeds and so
on. Liquid-liquid extraction is used in the extraction of fatty acids.
Factors controlling
the operation are:
 area of contact
between the streams,
time of contact,
properties of the
materials so far as the equilibrium distribution of the transferred component
is concerned, number
of contact stages employed.
In extraction from
a solid, the solid matrix may hinder diffusion and so control the rate
of extraction.
Rate of Extraction
The solution process
can be considered in terms of the usual rate equation
rate of solution = driving force/resistance.
In
this case, the driving force is the difference between the concentration
of the component being transferred, the solute, at the solid interface
and in the bulk of the solvent stream. For liquid-liquid extraction, a
double film must be considered, at the interface and in the bulk of the
other stream.
For solution from
a solid component, the equation can be written
dw/dt = KlA(ys
- y)
(9.8)
where
dw/dt is the rate of solution, Kl
is the mass-transfer coefficient, A is the interfacial area, and
ys and y, are the concentrations of the
soluble component in the bulk of the liquid and at the interface. It is
usually assumed that a saturated solution is formed at the interface and
ys is the concentration of a saturated solution at the
temperature of the system.
Examination
of eqn. (9.8) shows the effects of some of the factors, which can be used
to speed up rates of solution. Fine divisions of the solid component increases
the interfacial area A. Good mixing ensures that the local concentration
is equal to the mean bulk concentration. In other words, it means that
there are no local higher concentrations arising from bad stirring increasing
the value of y and so cutting down the rate of solution. An increase in
the temperature of the system will, in general, increase rates of solution
by not only increasing Kl, which is related to diffusion,
but also by increasing the solubility of the solute and so increasing
ys.
In
the simple case of extraction from a solid in a contact stage, a mass
balance on the solute gives the equation:
dw = Vdy
(9.9)
where V is
the quantity of liquid in the liquid stream.
Substituting for
dw in eqn. (9.8) we then have:
Vdy/dt = Kl A(ys
- y)
which
can then be integrated over time t during which time the concentration
goes from an initial value of y0 to a concentration y, giving
loge
[(ys - y0)/ (ys
- y)] = tKlA/V.
(9.10)
Equation
(9.10) shows, as might be expected, that the approach to equilibrium is
exponential with time. The equation cannot often be applied because of
the difficulty of knowing or measuring the interfacial area A.
In practice, suitable extraction times are generally arrived at by experimentation
under the particular conditions that are anticipated for the plant.
Stage-equilibrium Extraction
Analysis of an extraction operation depends upon establishing the equilibrium
and operating conditions. The equilibrium conditions are, in general,
simple. Considering the extraction of a solute from a solid matrix, it
is assumed that the whole of the solute is dissolved in the liquid in
one stage, which in effect accomplishes the desired separation. However,
it is not possible then to separate all of the liquid from the solid because
some solution is retained with the solid matrix and this solution contains
solute. As the solid retains solution with it, the content of solute in
this retained solution must be then progressively reduced by stage contacts.
For example, in the extraction of oil from soya bean seeds using hexane
or other hydrocarbon solvents, the solid beans matrix may retain its own
weight, or more, of the solution after settling. This retained solution
may therefore contain a substantial proportion of the oil. The equilibrium
conditions are simple because the concentration of the oil is the same
in the external solution that can be separated as it is in the solution
that remains with the seed matrix. Consequently, y, the concentration
of oil in the "light" liquid stream, is equal to x, the
concentration of oil in the solution in the "heavy" stream accompanying
the seed matrix. The equilibrium line is, therefore, plotted from the
relation y = x.
The
operating conditions can be analysed by writing mass balances round
the stages to give the eqn. (9.5). The plant is generally arranged in
the form of a series of mixers, followed by settlers in which the two
streams are separated prior to passing to the next stage of mixers. For
most purposes of analysis, the solid matrix need not be considered; the
solids can be thought of as just the means by which the two solution streams
are separated after each stage. So long as the same quantity of solid
material passes from stage to stage, and also the solids retain the same
quantity of liquid after each settling operation, the analysis is straightforward.
In eqn. (9.5), V refers to the liquid overflow stream from the
settlers, and L to the mixture of solid and solution that is settled
out and passes on with the underflow.
If
the underflow retains the same quantity of solution as it passes from
stage to stage, eqn. (9.5) simplifies to eqn. (9.6). The extraction operation
can then be analysed by application of step-by-step solution of the equations
for each stage, or by the use of the McCabe-Thiele graphical method.
 EXAMPLE
9.6. Counter current extraction of oil from soya beans with hexane
Oil is to be extracted from soya beans in a counter current stage-contact
extraction apparatus, using hexane. If the initial oil content of the
beans is 18%, the final extract solution is to contain 40% of oil, and
if 90% of the total oil is to be extracted, calculate the number of contact
stages that are necessary. Assume that the oil is extracted from the beans
in the first mixer, that equilibrium is reached in each stage, and that
the crushed bean solids in the underflow retain in addition half their
weight of solution after each settling stage.
The extraction plant is illustrated diagrammatically in Fig. 9.5.
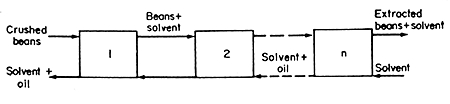
FIG. 9.5 Hexane extraction of oil from soya beans in stages
Each box represents a mixing-settling stage and the stages are numbered
from the stage at which the crushed beans enter.
The
underflow will be constant from stage to stage (a constant proportion
of solution is retained by the crushed beans) except for the first stage
in which the entering crushed beans (bean matrix and oil) are accompanied
by no solvent. After the first stage, the underflow is constant and so
all stages but the first can be treated by the use of eqn. (9.6).
To
illustrate the principles involved, the problem will be worked out from
stage-by-stage mass balances, and using the McCabe-Thiele graphical method.
Basis
for calculation: 100 kg raw material (bean solids and their associated
oil) entering stage 1. Concentrations of oil will be expressed as weight
fractions.
Overall mass
balance
In
100 kg raw material there will be 18% oil, that is 82 kg bean solids and
18 kg oil.
In the final underflow, 82 kg beans will retain 41 kg of solution, the
solution will contain 10% of the initial oil in the beans, that is, 1.8
kg so that there will be (18 - 1.8) = 16.2 kg of oil in the final overflow,
Extract contains
(16.2 x 60/40) = 24.3 kg of solvent
Total volume of final overflow = 16.2 + 24.3 = 40.5 kg
Total solvent entering = (39.2 + 24.3) = 63.5 kg
Note
that the solution passing as overflow between the stages is the same weight
as the solvent entering the whole system, i.e. 63.5 kg.
MASS BALANCE
Basis: 100 kg beans
| Mass
in |
(kg)
|
 |
Mass
out |
(
kg) |
| Underflow |
|
|
Underflow
|
|
| Raw beans |
100.0 |
|
Extracted beans
+ solution |
123.0 |
|
Bean solids |
82.0 |
|
Bean solids |
82.0 |
| Oil
|
18.0 |
|
Oil |
1.8 |
| |
|
|
Solvent |
39.2 |
| Overflow |
|
|
Overflow |
|
| Solvent |
63.5 |
|
Total extract |
40.5 |
| |
|
|
Solvent |
24.3 |
| |
|
|
Oil |
16.2 |
| |
|
|
|
|
| Total |
163.5 |
|
Total |
163.5 |
Analysis of stage 1
Oil
concentration in underflow = product concentration = 0.4.
It is an equilibrium stage, so oil concentration in underflow equals oil
concentration in overflow. Let y2 represent the concentration
of oil in the overflow from stage 2 passing in to stage 1. Then oil entering
stage 1 equals oil leaving stage 1.
Therefore balance on oil:
63.5y2 + 18 = 41 x 0.4 + 40.5 x 0.4
y2 = 0.23.
Analysis
of stage 2
x2 = y2 = 0.23
Therefore balance on oil:
41
x 0.4 + 63.5y3 = 63.5 x 0.23 + 41 x 0.23,
y3 = 0.12.
Analysis
of stage 3
x3 = y3=
0.12
Therefore balance on oil:
41 x 0.23 + 63.5y4
= 63.5 x 0.12 + 41 x 0.12
y4= 0.049
Analysis
of stage 4
x4
= y4 = 0.049,
Therefore balance
on oil
41 x 0.12 + 63.5 y5 = 63.5 x 0.049 + 41 x 0.049,
y5 = 0.00315.
The
required terminal condition is that the underflow from the final nth
stage will have less than 1.8 kg of oil, that is, that xn
is less than 1.8/41 = 0.044.
Since xn = yn and we have calculated that y5
is 0.00315 which is less than 0.044 (whereas y4 = 0.049
was not), four stages of contact will be sufficient for the requirements
of the process.
Using the graphical
method, the general eqn. (9.6) can be applied to all stages after the
first.
From
the calculations above for the first stage, we have x1
= 0.4, y2 = 0.23 and these can be considered as the
entry conditions xa and ya for the
series of subsequent stages.
Applying eqn. (9.6)
the operating line equation:
yn+1
= xnL/V + ya - xaL/V
Now L = 41, V = 63.5, ya = 0.23 xa
= 0.4
yn+1
= 0.646 xn - 0.028
The equilibrium line
is:
yn = xn
The operating line
and the equilibrium line have been plotted on Fig. 9.6.
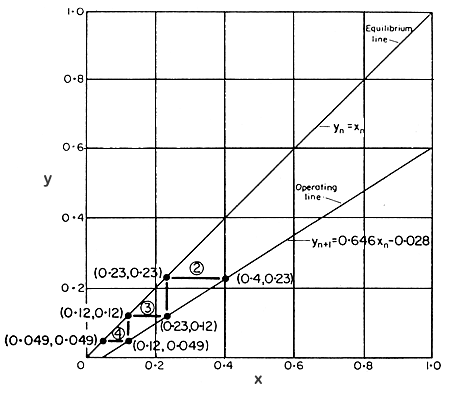
FIG. 9.6 Hexane extraction of oil from soyabeans: McCabe-Thiele plot
The
McCabe-Thiele construction has been applied, starting with the entry conditions
to stage 2 (stage 1 being the initial mixing stage) on the operating line,
and it can be seen that three steps are not sufficient, but that four
steps give more than the minimum separation required. Since the initial
stage is included but not shown on the diagram, four stages are necessary,
which is the same result as was obtained from the step-by-step calculations.
The
step construction on the McCabe-Thiele diagram can also be started from
the nth stage, since we know that yn+1 which is the
entering fresh solvent, equals 0. This will also give the same number
of stages, but it will apparently show slightly different stage concentrations.
The apparent discrepancy arises from the fact that in the overall balance,
a final (given) concentration of oil in the overflow stream of 0.044 was
used, and both the step-by-step equations and the McCabe-Thiele operating
line depend upon this. In fact, this concentration can never be reached
using a whole number of steps under the conditions of the problem and
to refine the calculation it would be necessary to use trial-and-error
methods. However, the above method is a sufficiently close approximation
for most purposes.
In some practical extraction applications, the solids may retain different
quantities of the solvent in some stages of the plant. For example, this
might be due to rising concentrations of extract having higher viscosities.
In this case, the operating line is not straight, but step-by-step methods
can still be used. For some of the more complex situations other graphical
methods using triangular diagrams can be employed and a discussion of
their use may be found in Charm (1970), Coulson
and Richardson (1978) or Treybal (1970).
It
should be noted that in the chemical engineering literature what has here
been called extraction is more often called leaching, the term extraction
being reserved for liquid-liquid contacting using immiscible liquids.
"Extraction" is, however, in quite general use in the food industry
to describe processes such as the one in the above example, whereas the
term "leaching" would probably only cause confusion.
Washing
Washing is almost identical to extraction, the main distinction being
one of the emphasis in that in washing the inert material is the required
product, and the solvent used is water which is cheap and readily available.
Various washing situations are encountered and can be analysed. That to
be considered is one in which a solid precipitate, the product, retains
water which also contains residues of the mother liquor so that on drying
without washing these residues will remain with the product. The washing
is designed to remove them, and examples are butter and casein and cheese
washing in the dairy industry.
Calculations
on counter current washing can be carried out using the same methods as
discussed under extraction, working from the operating and equilibrium
conditions. In washing, fresh water is often used for each stage and the
calculations for this are also straightforward.
In
multiple washing, the water content of the material is xw
(weight fraction) and a fraction of this, x, is impurity, and to
this is added yxw of wash water, and after washing thoroughly,
the material is allowed to drain. After draining it retains the same quantity,
approximately, of water as before, xw. The residual
yxw of wash liquid, now at equilibrium containing the same
concentration of impurities as in the liquid remaining with the solid,
runs to waste. Of course in situations in which water is scarce counter
current washing may be worthwhile.
The
impurity which was formerly contained in xw of water
is now in a mass (xw + yxw): its concentration
x has fallen by the ratio of these volumes, that is to x
[xw/(xw + yxw)].
So the concentration
remaining with the solid after one washing, x1, is given
by:
x1
= x[xw /xw(1 + y)]
= x[1/(1 + y)]
after two washings:
x2 = x1[1/(1 + y)] = x[1/(1
+ y)]2
and so after n
washings:
xn = x[1/(1 + y)]n
(9.11)
If,
on the other hand, the material is washed with the same total quantity
of water as in the n washing stages, that is nyxw, but all
in one stage, the impurity content will be:
x'n
= x[1/(ny + 1)]
(9.12)
and
it is clear that the multiple contact washing is very much more efficient
in reducing the impurity content that is single contact washing, both
using the same total quantity of water.
EXAMPLE
9.7. Washing of casein curd
After precipitation and draining procedures, it is found that 100 kg of
fresh casein curd has a liquid content of 66% and this liquid contains
4.5% of lactose. The curd is washed three times with 194 kg of fresh water
each time. Calculate the residual lactose in the casein after drying.
Also calculate the quantity of water that would have to be used in a single
wash to attain the same lactose content in the curd as obtained after
three washings. Assume perfect washing, and draining of curd to 66% of
moisture each time.
100 kg of curd contain
66 kg solution. The 66 kg of solution contain 4.5% that is 3 kg of lactose.
In the first wash
(194 + 66) = 260 kg of solution contain 3 kg lactose.
In 66 kg of solution remaining there will be (66/260) x 3 = 0.76 kg of
lactose.
After the second
wash the lactose remaining will be (66/260) x 0.76 = 0.19kg
After the third wash
the lactose remaining will be (66/260) x 0.19 = 0.048 kg
Or, after three washings
lactose remaining will be 3 x (66/260)3 =
0.048 kg as above
So, after washing
and drying 0.048 kg of lactose will remain with 34 kg dry casein so that
lactose content
of the product = 0.048/34.05
= 0.14%
and total wash water
= 3 x 194 = 582 kg
To reduce the impurity
content to 0.048 kg in one wash would require x kg of water, where
(3 x 66)/(x + 66) = 0.048 kg
x = 4060 kg
and so the total wash water
= 4060 kg
Alternatively using
eqns. (9.11) and (9.12)
xn = x[1/(1 + y)]n
= 3[1/(1 + 194/66)]3
= 0.049
xn'
= x[1/(ny + 1)]
0.049 = 3[1/(ny+
1)]
ny = 61.5.
Total wash water
= nyxw = 61.5 x 66
= 4060 kg.
Extraction and Washing Equipment
The
first stage in an extraction process is generally mechanical grinding,
in which the raw material is shredded, ground or pressed into suitably
small pieces or flakes to give a large contact area for the extraction.
In some instances, for example in sugar-cane processing and in the extraction
of vegetable oils, a substantial proportion of the desired products can
be removed directly by expression at this stage and then the remaining
solids are passed to the extraction plant. Fluid solvents are easy to
pump and so overflows are often easier to handle than underflows and sometimes
the solids may be left and solvent from successive stages brought to them.
This
is the case in the conventional extraction battery. In this a number
of tanks, each suitable both for mixing and for settling, are arranged
in a row or a ring. The solids remain in the one mixer-settler and the
solvent is moved progressively round the ring of tanks, the number, n,
often being about 12. At any time, two of the tanks are out of operation,
one being emptied and the other being filled. In the remaining (n
- 2), tanks extraction is proceeding with the extracting liquid solvent,
usually water, being passed through the tanks in sequence, the "oldest"
(most highly extracted) tank receiving the fresh liquid and the "youngest"
(newly filled with fresh raw material) tank receiving the most concentrated
liquid. After leaving this "youngest" tank, the concentrated
liquid passes from the extraction battery to the next stage of the process.
After a suitable interval, the connections are altered so that the tank
which has just been filled becomes the new “youngest" tank.
The former "oldest" tank comes out of the sequence and is emptied,
the one that was being emptied is filled and the remaining tanks retain
their sequence but with each becoming one stage "older". This
procedure which is illustrated in Fig. 9.7 in effect
accomplishes counter current extraction, but with only the liquid physically
having to be moved apart from the emptying and filling in the terminal
tanks.
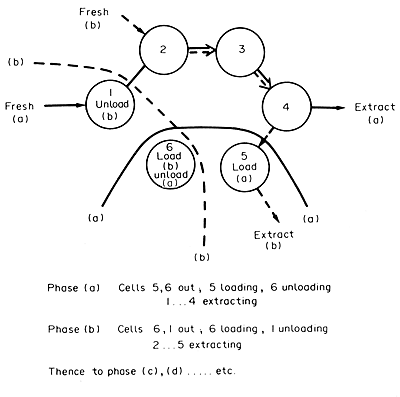
FIG. 9.7 Extraction battery
In the same way and for the same reasons as with counter flow heat exchangers,
this counter current (or counter flow) extraction system provides the
maximum mean driving force, the log mean concentration difference in this
case, contrasting with the log mean temperature difference in the heat
exchanger. This ensures that the equipment is used efficiently.
In
some other extractors, the solids are placed in a vertical bucket conveyor
and moved up through a tower down which a stream of solvent flows. Other
forms of conveyor may also be used, such as screws or metal bands, to
move the solids against the solvent flow. Sometimes centrifugal forces
are used for conveying, or for separating after contacting.
Washing
is generally carried out in equipment that allows flushing of fresh water
over the material to be washed. In some cases, the washing is carried
out in a series of stages. Although water is cheap, in many cases very
large quantities are used for washing so that attention paid to more efficient
washing methods may well be worthwhile. Much mechanical ingenuity has
been expended upon equipment for washing and many types of washers are
described in the literature.
 Contact-Equilibrium
Processes - APPLICATIONS > CRYSTALLIZATION
 Back
to the top |
