The
flow will continue until the concentration rises to the point where
its osmotic pressure
equals the applied pressure. Such a process is called reverse osmosis
and special artificial membranes have been made with the required "tight" structure
to retain all but the smallest molecules such as those of water.
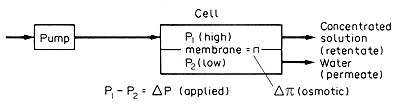
Figure 9.9
Reverse osmosis systems
Also, "looser" membranes have been developed through which not
only water, but also larger solute molecules can selectively pass if driven
by imposed pressure. Membranes are available which can retain large molecules,
such as proteins, while allowing through smaller molecules. Because the
larger molecules are normally at low molar concentrations, they exert
very small osmotic pressures, which therefore enter hardly at all into
the situation. Following the resemblance to conventional filtration, this
process is called ultrafiltration. In general, ultrafiltration
needs relatively low differential pressures, up to a few atmospheres.
If higher pressures are used, a protein or solute gel appears to form
on the membrane, which resists flow, and so the increased pressure may
not increase the transfer rate.
Important
applications for ultrafiltration are for concentrating solutions of large
polymeric molecules, such as milk and blood proteins. Another significant
application is to the concentration of whey proteins. Reverse osmosis,
on the other hand, is concerned mainly with solutions containing smaller
molecules such as simple sugars and salts at higher molar concentrations,
which exert higher osmotic pressures. To overcome these osmotic pressures,
high external pressures have to be exerted, up to the order of 100 atmospheres.
Limitations to increased flow rates arise in this case from the mechanical
weaknesses of the membrane and from concentration of solutes which causes
substantial osmotic "back" pressure. Applications in the food
industry are in separating water from, and thus concentrating, solutions
such as fruit juices.
Rate of Flow Through Membranes
There are various
equations to predict the osmotic pressures of solutions, perhaps the best
known being the van't Hoff equation:
P
= MRT
(9.17)
in
which P
(pi) is the osmotic pressure (kPa), M the molar concentration (moles
m-3), T the absolute temperature (°K), and
R the universal gas a constant . This equation is only strictly
accurate when the dilution is infinitely great, but it can still be used
as an approximation at higher concentrations.
The net driving force
for reverse osmosis is then the difference between the applied differential
pressure DP,
and the differential osmotic pressure, DP ,
which resists the flow in the desired "reverse" direction.
Therefore it can be described by the standard rate equation, with the
rate of mass transfer
being equal to the driving force multiplied by the appropriate mass-transfer
coefficient:
dw/dt = KA[DP - DP]
(9.18)
where
dw/dt is the rate of mass transfer, K is the mass
transfer coefficient, A the area through which the transfer is
taking place, DP
is the net applied pressure developed across the membrane and DP
is the net osmotic pressure across the membrane and resisting the flow.
DP
is therefore the difference in the applied pressure on the solutions at
each side of the membrane and DP
is the difference in the osmotic pressures of the two solutions, as in
Fig. 9.9. The gas constant is 8.314 kPa
m
 EXAMPLE
9.11. Reverse osmosis: concentration of sucrose solution by reverse
osmosis
A solution of sucrose in water at 25°C is to be concentrated by reverse
osmosis. It is found that, with a differential applied pressure of 5000
kPa,
the rate of movement of the water molecules through the membrane is 25
kg m-2 h-1 for a 10% solution of sucrose. Estimate
the flow rate through
the membrane for a differential pressure of 10,000 kPa with the 10% sucrose
solution, and also estimate the flow rate for a differential pressure
of 10,000 kPa but with a sucrose concentration of 20%.
For sucrose, the
molecular weight is 342 so for a 10% solution, molar concentration (from
tables such as Perry, 1997) is 0.304 moles m-3 and for 20%,
0.632 moles m-3.
Applying eqn. (9.17):
For 10% solution
P =
0.304 x 8.314 x 298
=
753 kPa
For 20% solution P =
0.632 x 8.314 x 298
=
1566 kPa.
So we have for the
first case, for 1 m2 of membrane:
dw/dt =
25 = K[5000 - 753]
K =
5.9 x 10-3 kg m-2 h-1 kPa-1
So
for DP = 10,000 kPa,
dw/dt =
5.9 x 10-3[10,000 - 753]
=
55 kg m-2 h-1
And
for DP =
10,000 and 20% soln.
dw/dt =
5.9 x 10-3[10,000 - 1566]
=
50 kg m-2 h-1
Experimental values
of the osmotic pressure of the sucrose solutions at 10% and 20% were measured
to be 820 and 1900 kPa respectively, demonstrating the relatively small
error arising from applying the van't Hoff equation to these quite highly
concentrated solutions. Using these experimental values slightly reduces
the predicted flow as can be seen by substituting in the equations.
In
ultrafiltration practice, it is found that eqn. (9.18) applies only for
a limited time and over a limited range of pressures. As pressure increases
further, the flow ceases to rise, or even falls. This appears to be caused,
in the case of ultrafiltration, by increased mechanical resistance at
the surface of the membrane due to the build-up of molecules forming a
layer which is like a gel and which resists flow through it. Under these
circumstances, flow is better described by diffusion equations
through this resistant layer leading to equation:
dw/dt = K’ A loge(ci /cb) (9.19)
where
ci and cb are the solute concentrations
at the interface and in the bulk solution respectively. The effect of
the physical properties of the material can be predicted from known relationships
for the mass transfer coefficient K' (m s-1), which
can be set equal to D/d
where D is the diffusivity of the solute (m2 s-1),
divided by d,
the thickness of the gel layer (m). This equation has been found to predict,
with reasonable accuracy, the effect on the mass transfer of changes in
the physicochemical properties of the solution. This is done through well
established relationships between the diffusivity D, the mass transfer
coefficient K', and other properties such as density (r),
viscosity (m)
and temperature (T) giving:
(K'd/D) = a[(dvr/m)m]
x (m/rD)n
or
(Sh) = (K'd/D) = a(Re)m(Sc)n
(9.20)
where
d is the hydraulic diameter, (Sh) the Sherwood number
(K'd/D); (Sc) the Schmidt number (m/rD);
and a, m, n are constants. Notice the similar form of eqn. (9.20) and
the equation for heat transfer in forced convection, (Nu) = a'(Re)m'(Pr)n',
with (Sh) replacing (Nu) and (Sc) replacing (Pr). This is another aspect
of the similarity between the various transport phenomena. These ideas,
and the uses that can be made of them, are discussed in various books,
such as Coulson and Richardson (1977) and
McCabe and Smith (1975), and more comprehensively
in Bird, Stewart and Lightfoot (1960).
In the case of reverse
osmosis, the main resistance arises from increased concentrations and
therefore increased back pressure from the osmotic forces. The flow rate
cannot be increased by increasing the pressure because of the limited
strength of membranes and their supports, and the difficulties of designing
and operating pumps for very high pressures.
Membrane Equipment
The
equipment for these membrane separation processes consists of the necessary
pumps, flow systems and membranes. In the case of ultrafiltration, the
membranes are set up in a wide variety of geometrical arrangements, mostly
tubular but sometimes in plates, which can be mounted similarly to a filter
press or plate heat exchanger. Flow rates are kept high over the surfaces
and recirculation of the fluid on the high pressure, or retentate, side
is often used; the fluid passing through, called the permeate, is usually
collected in suitable troughs or tanks at atmospheric pressure.
In
the case of reverse osmosis, the high pressures dictate mechanical
strength, and stacks of
flat disc membranes can be used one above the other. Another system
uses very small diameter (around 0.04 mm) hollow filaments on plastic
supports;
the diameters are small to provide strength but preclude many food
solutions because of this very small size. The main flow in reverse
osmosis is the
permeate.
The systems can be
designed either as continuous or as batch operations. One limitation to
extended operation arises from the need to control growth of bacteria.
After a time bacterial concentrations in the system, for example in the
gel at the surface of the ultrafiltration membranes, can grow so high
that cleaning must be provided. This can be difficult as many of the membranes
are not very robust either to mechanical disturbance or to the extremes
of pH which could give quicker and better cleaning.
EXAMPLE 9.12. Ultrafiltration of whey
It is desired to increase the protein concentration in whey, from cheese
manufacture, by a factor of 12 by the use of ultrafiltration to give
an
enriched fraction which can subsequently be dried and used to produce
a 50% protein whey powder. The whey initially contains 6% of total
solids,
12% of these being protein. Pilot scale measurements on this whey
show that a permeate flow of 30 kg m-2 h-1 can
be expected, so that if the plant
requirement is to handle 30,000 kg in 6 hours, estimate the area
of membrane needed. Assume that the membrane rejection of the protein
is over 99%,
and calculate the membrane rejection of the non-protein constituents.
Protein in initial whey = 6 x 0.12 = 0.72%
Protein in retentate = 12 x concentration in whey
=
12 x 6 x 0.12
=
8.6%.
Setting
out a mass balance, basis 100 kg whey:
| |
Water |
Protein |
Non-protein |
| |
(kg) |
(kg) |
(kg) |
| Initial
whey |
94.0 |
0.7 |
5.3 |
| Retentate |
6.7 |
0.7 |
0.7 |
| Permeate |
87.3 |
0.0 |
4.6 |
Water
removed per 100 kg whey =
87.3 kg
The equipment has
to process 30,000 kg in 6 h so the membrane has to pass the permeate
at:
(30,000 x 91.9)/(100 x 6) = 4595 kg h-1
and permeate filtration rate is 30 kg m-2 h-1
Therefore required area of membrane = 4595/30
=
153 m2
Non-protein rejection rate =
0.7/5.3
=
13%
Membrane
processes generally use only one apparent contact stage, but product accumulation
with time, or with progression through a flow unit, gives situations which
are equivalent to multistage units. Dialysis, which is a widely
used laboratory membrane-processing technique, with applications in industry,
sometimes is operated with multiple stages.
These membrane concentration and separation processes have great potential
advantages in the simplicity of their operation and because drastic conditions,
in particular the use of heat leading to thermal degradation, are not
involved. Therefore more extensive application can be expected as membranes,
flow systems and pumps are improved. Discussion of these processes can
be found in papers by Thijssen
(1974) and in Sourirajan (1977).
 Contact-Equilibrium
Processes - APPLICATIONS > DISTILLATION
 Back
to the top |
Rate
of Flow Through Membranes