Unit Operations in Food Processing

In a series of contact stages, in which the components counter flow from one stage to another, mass balances can be written around any stage, or any number of stages. This enables operating equations to be set down to connect the flow rates and the compositions of the streams. Consider the generalized system shown in Fig. 9.2, in which there is a stage contact process operating with a number of stages and two contacting streams. By convention, the mass flow of the light stream is denoted by V and the flow of the heavy stream by L.as shown in Figure 9.2 (left hand side)

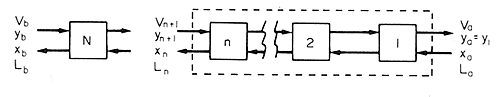
Figure 9.2 Contact equilibrium stages

Taking a mass balance over the first n stages as shown in Figure 9.2 (right hand side) we can write, for the total flow, mass entering must equal mass leaving, so:

V_n+1 + L_a = V_a + L_n

and for the component being exchanged:

V_n+1 y_n+1 + L_ax_a= V_ay_a + L_nx_n

where V is the mass flow rate of the light stream, L is the flow rate of the heavy stream, y is the concentration of the component being exchanged in the light stream and x is the concentration of the component being exchanged in the heavy stream. In the case of the subscripts, n denotes conditions at equilibrium in the nth stage, n + 1 denotes conditions at equilibrium in the (n + 1)th stage and a denotes the conditions of the streams entering and leaving stage 1, one being raw material and one product from that stage

Eliminating V_n+1 between these equations, we have:

V_n+1 = L_n - L_a + V_a
and so,

y_n+1(L_n - L_a + V_a ) = V_ay_a + L_nx_n - L_ax_a

y_n+1 = x_n [L_n / (L_n - L_a+ V_a)] + [(V_ay_a - L_ax_a)/ (L_n - L_a + V_a)] (9.5)

This is an important equation as it expresses the concentration in one stream in the (n + 1)th stage in terms of the concentration in the other streams in the nth stage. In many practical cases in which equal quantities, or equal molar quantities, of the carrying streams move from one stage to another, that is where the flow rates are the same in all contact stages, then for:

lighter phase L_n+1 = L_n = ... L_a = L
heavier phase V_n+1 =… = V_a. = V

A simplified equation can be written for such cases:
y_n+1 = x_nL / V + y_a - x_aL / V (9.6)

Contact-Equilibrium Processes - THEORY > CALCULATION OF SEPARATION IN CONTACT-EQUILIBRIUM PROCESSES

Back to the top