This
consists of a continuous pipe that changes its diameter, passing into
and out of a unit of processing plant, which is represented by a tank.
The processing equipment might be, for example, a pasteurizing heat exchanger.
Also in the system is a pump to provide the energy to move the fluid.
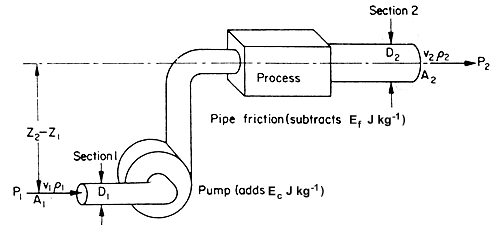
Fiigure 3.3. Mass and energy balance in fluid flow
In
the flow system of Fig. 3.3 we can apply the law of conservation
of mass to obtain a mass balance. Once the system is working steadily,
and if there is no accumulation of fluid in any part the system, the quantity
of fluid that goes in at section 1 must come out at section 2. If the
area of the pipe at section 1 is A1 , the velocity at
this section, v1 and the fluid density r1,
and if the corresponding values at section 2 are A2,
v2,
r2,
the mass balance can be expressed as
r1A1v1
= r2A2v2
(3.4)
If the fluid is incompressible
r1
= r2
so in this case
A1v1 = A2v2
(3.5)
Equation
(3.5) is known as the continuity equation for liquids and is frequently
used in solving flow problems. It can also be used in many cases of gas
flow in which the change in pressure is very small compared with the system
pressure, such as in many air-ducting systems, without any serious error.
 EXAMPLE
3.4. Velocities of flow
Whole milk is flowing into a centrifuge through a full 5 cm diameter pipe
at a velocity of 0.22 m s-1, and in the centrifuge it is separated
into cream of specific gravity 1.01 and skim milk of specific gravity
1.04. Calculate the velocities of flow of milk and of the cream if they
are discharged through 2 cm diameter pipes. The specific gravity of whole
milk of 1.035.
From eqn. (3.4):
r1A1v1
= r2A2v2
+ r3A3v3
where
suffixes 1, 2, 3 denote respectively raw milk, skim milk and cream. Also,
since volumes will be conserved, the total leaving volumes will equal
the total entering volume and so
A1v1 = A2v2
+ A3v3 and from this equation
v2 = (A1v1 - A3v3)/A2
(a)
This expression can
be substituted for v2 in the mass balance equation to
give:
r1A1v1
= r2A2(A1v1
– A3v3)/A2
+ r3A3v3
r1A1v1
= r2A1v1
- r2A3v3
+ r3A3v3.
So A1v1(r1
- r2)
= A3v3(r3
- r2)
(b)
From the known facts
of the problem we have:
A1 = (p/4)
x (0.05)2 = 1.96 x 10-3 m2
A2 = A3 = (p/4)
x (0.02)2 = 3.14 x 10-4 m2
v1 = 0.22
m s-1
r1
= 1.035 x rw,
r2
= 1.04 x rw
, r3
= 1.01 x rw
where rw
is the density of water.
Substituting these
values in eqn. (b) above we obtain:
-1.96 x 10-3
x 0.22 (0.005) = -3.14 x 10-4 x v3 x
(0.03)
so
v3
= 0.23 m s-1
Also from eqn. (a)
we then have, substituting 0.23 m s-1 for v3,
v2 = [(1.96 x 10-3 x 0.22) - (3.14 x 10-4
x 0.23)] / 3.14 x 10-4
= 1.1m
s-1
Energy Balance
In
addition to the mass balance, the other important quantity we must consider
in the analysis of fluid flow, is the energy balance. Referring again
to Fig. 3.3, we shall consider the changes in the total energy
of unit mass of fluid, one kilogram, between Section 1 and Section 2.
Firstly,
there are the changes in the intrinsic energy of the fluid itself which
include changes in:
(1) Potential energy.
(2) Kinetic energy.
(3) Pressure energy.
Secondly, there may be energy interchange with the surroundings including:
(4) Energy lost to the surroundings due to friction.
(5) Mechanical energy added by pumps.
(6) Heat energy in heating or cooling the fluid.
In the analysis of the energy balance, it must be remembered that energies
are normally measured from a datum or reference level. Datum levels may
be selected arbitrarily, but in most cases the choice of a convenient
datum can be made readily with regard to the circumstances.
Potential energy
Fluid
maintained above the datum level can perform work in returning to the
datum level. The quantity of work it can perform is calculated from the
product of the distance moved and the force resisting movement; in this
case the force of gravity. This quantity of work is called the potential
energy of the fluid.
Thus the potential energy of one kilogram of fluid at a height of Z
(m) above its datum is given by Ep, where
Ep
= Zg (J)
Kinetic energy
Fluid
that is in motion can perform work in coming to rest. This is equal to
the work required to bring a body from rest up to the same velocity, which
can be calculated from the basic equation
v2
= 2as, therefore s = v2/2a,
where v (m
s-1) is the final velocity of the body, a (m s-2)
is the acceleration and s (m) is the distance the body has moved.
Also work done =
W = F x s, and from Newton's Second Law, for m kg of
fluid
F = ma
and so Ek
= W = mas = mav2/2a = mv2/2
The energy of motion,
or kinetic energy, for 1 kg of fluid is therefore given by Ek
where
Ek
= v2/2 (J).
Pressure energy
Fluids exert a pressure on their surroundings. If the volume of a fluid
is decreased, the pressure exerts a force that must be overcome and so
work must be done in compressing the fluid. Conversely, fluids under pressure
can do work as the pressure is released. If the fluid is considered as
being in a cylinder of cross-sectional area A (m2) and
a piston is moved a distance L (m) by the fluid against the pressure
P (Pa) the work done is PAL joules. The quantity of the
fluid performing this work is ALr
(kg). Therefore the pressure energy that can be obtained from one kg of
fluid (that is the work that can be done by this kg of fluid) is given
by Er where
Er
= PAL / ALr
= P/r
(J)
Friction loss
When a fluid moves through a pipe or through fittings, it encounters frictional
resistance and energy can only come from energy contained in the fluid
and so frictional losses provide a drain on the energy resources of the
fluid. The actual magnitude of the losses depends upon the nature of the
flow and of the system through which the flow takes place. In the system
of Fig. 3.3, let the energy lost by 1 kg fluid between section
1 and section 2, due to friction, be equal to Eƒ
(J).
Mechanical energy
If there is a machine putting energy into the fluid stream, such as a
pump as in the system of Fig. 3.3, the mechanical energy added
by the pump per kg of fluid must be taken into account. Let the pump energy
added to 1 kg fluid be Ec (J). In some cases a machine
may extract energy from the fluid, such as in the case of a water turbine.
Other effects
Heat might be added or subtracted in heating or cooling processes, in
which case the mechanical equivalent of this heat would require to be
included in the balance. Compressibility terms might also occur, particularly
with gases, but when dealing with low pressures only they can usually
be ignored.
For the present let
us assume that the only energy terms to be considered are Ep,
Ek, Er, Ef, Ec.
Bernouilli's Equation
We are now in a position to write the energy balance for the fluid between
section 1 and section 2 of Fig. 3.3.
The total energy of one kg of fluid entering at section 1 is equal to
the total energy of one kg of fluid leaving at section 2, less the energy
added by the pump, plus friction energy lost in travelling between the
two sections. Using the subscripts 1 and 2 to denote conditions at section
1 or section 2, respectively, we can write
Ep1
+ Ek1 + Er1
= Ep2 + Ek2
+ Er2 + Ef - Ec.
(3.6.)
Therefore Z1g + v12/2
+ P1/r1
= Z2g + v22/2
+ P2/r2
+ Ef - Ec.
(3.7)
In the special case
where no mechanical energy is added and for a frictionless fluid,
Ec = Ef = 0, and we have
Z1g
+ v12/2 + P1/r1
= Z2g + v22/2
+ P2/r2
(3.8)
and since this is
true for any sections of the pipe the equation can also be written
Zg + v2/2 + P/r
= k
(3.9)
where k is a constant.
Equation
(3.9) is known as Bernouilli's equation. First discovered by the
Swiss mathematician Bernouilli in 1738, it is one of the foundations of
fluid mechanics. It is a mathematical expression, for fluid flow, of the
principle of conservation of energy and it covers many situations of practical
importance.
Application
of the equations of continuity, eqn. (3.4) or eqn. (3.5), which represent
the mass balance, and eqn. (3.7) or eqn. (3.9), which represent the energy
balance, are the basis for the solution of many flow problems for fluids.
In fact much of the remainder of this chapter will be concerned with applying
one or another aspect of these equations.
The
Bernouilli equation is of sufficient importance to deserve some further
discussion. In the form in which it has been written in eqn. (3.9) it
will be noticed that the various quantities are in terms of energies per
unit mass of the fluid flowing. If the density of the fluid flowing multiplies
both sides of the equation, then we have pressure terms and the equation
becomes:
rZg
+ rv2/2
+ P = k'
(3.10)
and
the respective terms are known as the potential head pressure, the velocity
pressure and the static pressure.
On
the other hand, if the equation is divided by the acceleration due to
gravity, g, then we have an expression in terms of the head of
the fluid flowing and the equation becomes:
Z + v2/2g
+ P/rg
= k''
(3.11)
and
the respective terms are known as the potential head, the velocity head
and the pressure head.
The most convenient form for the equation is chosen for each particular
case, but it is important to be consistent having made a choice.
If
there is a constriction in a pipe and the static pressures are measured
upstream or downstream of the constriction and in the constriction itself,
then the Bernouilli equation can be used to calculate the rate of flow
of the fluid in the pipe. This assumes that the flow areas of the pipe
and in the constriction are known. Consider the case in which a fluid
is flowing through a horizontal pipe of cross-sectional area A1
and then it passes to a section of the pipe in which the area is reduced
to A2. From the continuity equation [eqn. (3.5)] assuming
that the fluid is incompressible:
A1v1 = A2v2
and so
v2 =
v1A1 /A2
Since the pipe is
horizontal
Z1 = Z2
Substituting in eqn.
(3.8)
v12/2
+ P1 /r1
= v12 A12
/(2 A22) + P2
/r2
and since r1
= r2
as it is the same fluid throughout and it is incompressible,
P1
- P2
= r1
v12((A12 /A22)
- 1)/2.
(3.12)
From
eqn. (3.12), knowing P1, P2, A1,
A2, r1,
the unknown velocity in the pipe, v1, can be calculated.
Another
application of the Bernouilli equation is to calculate the rate of flow
from a nozzle with a known pressure differential. Consider a nozzle
placed in the side of a tank in which the surface of the fluid in the
tank is Z ft above the centre line of the nozzle as illustrated in Fig.
3.4.
FIG. 3.4. Flow from a nozzle.
Take
the datum as the centre of the nozzle. The velocity of the fluid entering
the nozzle is approximately zero, as the tank is large compared with the
nozzle. The pressure of the fluid entering the nozzle is P1
and the density of the fluid r1.
The velocity of the fluid flowing from the nozzle is v2
and the pressure at the nozzle exit is 0 as the nozzle is discharging
into air at the datum pressure. There is no change in potential energy
as the fluid enters and leaves the nozzle at the same level. Writing the
Bernouilli equation for fluid passing through the nozzle:
0 + 0 + P1
/r1
= 0 + v22/2 + 0
v22 = 2 P1 /r1
whence
but
P1
/r1
= gZ
(where Z is the head of fluid above the nozzle) therefore
EXAMPLE
3.5. Pressure in a pipe
Water flows at the rate of 0.4 m3 min-1 in a 7.5
cm diameter pipe at a pressure of 70 kPa. If the pipe reduces to 5 cm
diameter calculate the new pressure in the pipe.
Density of water is 1000 kg m-3.
Flow rate of water = 0.4 m3 min-1 = 0.4/60 m3
s-1.
Area of 7.5 cm
diameter pipe = (p/4)D2
= (p/4)(0.075)2
= 4.42 x 10-3 m2.
So velocity of flow in 7.5 cm diameter pipe,
v1 = (0.4/60)/(4.42 x 10-3) = 1.51 m s-1
Area of 5 cm diameter
pipe = (p/4)(0.05)2
= 1.96 x 10-3 m2
and so velocity
of flow in 5 cm diameter pipe,
v2 = (0.4/60)/(1.96 x 10-3) = 3.4 m s-1
Now
Z1g
+ v12/2
+ P1 /r1
= Z2g
+ v22/2
+ P2 /r2
and so
0 + (1.51)2/2 + 70 x 103/1000 = 0 + (3.4)2/2
+ P2/1000
0 + 1.1 + 70 = 0 + 5.8 + P2/1000
P2/1000 =
(71.1 - 5.8) = 65.3
P2 = 65.3k Pa.
EXAMPLE
3.6. Flow rate of olive oil
Olive oil of specific gravity 0.92 is flowing in a pipe of 2 cm diameter.
Calculate the flow rate of the olive oil, if an orifice is placed in the
pipe so that the diameter of the pipe in the constriction is reduced to
1.2 cm, and if the measured pressure difference between the clear pipe
and the most constricted part of the pipe is 8 cm of water.
Diameter of pipe, in clear section, equals 2 cm and at constriction equals
1.2 cm.
A1/A2
= (D1/D2)2 = (2/1.2)2
Differential head = 8 cm water.
Differential pressure =
Zrg
= 0.08 x 1000 x 9.81 = 785 Pa.
substituting in eqn.
(3.12)
785
= 0.92 x 1000 x v2 [(2/1.2)4 - 1 ] /2
v2 = 785/3091
v = 0.5 m s-1
EXAMPLE
3.7. Mass flow rate from a tank
The level of water in a storage tank is 4.7 m above the exit pipe. The
tank is at atmospheric pressure and the exit pipe discharges into the
air. If the diameter of the exit pipe is 1.2 cm what is the mass rate
of flow through this pipe?
From eqn. (3.13)
 |
= 9.6
m s-1. |
Now area of pipe, A
= (p/4)D2
= (p/4)
x (0.012)2
= 1.13 x 10-4 m2
Volumetric flow
rate, Av
= 1.13 x 10-4
m2 x 9.6 m s-1
= 1.13 x 10-4 x 9.6 m3 s-1
= 1.08 x 10-3 m3 s-1
Mass flow rate, rAv
= 1000 kg m-3
x 1.08 x 10-3 m3 s-1
= 1.08 kg s-1
EXAMPLE
3.8. Pump horsepower
Water is raised from a reservoir up 35 m to a storage tank through a 7.5
cm diameter pipe. If it is required to raise 1.6 cubic metres of water
per minute, calculate the horsepower input to a pump assuming that the
pump is 100% efficient and that there is no friction loss in the pipe.
1 Horsepower = 0.746 kW.
Volume of flow, V
= 1.6 m3
min-1 = 1.6/60 m3 s-1 = 2.7 x 10-2
m3 s-1
Area of pipe, A
= (p/4)
x (0.075)2 = 4.42 x 10-3 m2,
Velocity in pipe,
v
= 2.7 x 10-2/(4.42
x 10-3) = 6 m s-1,
And so applying
eqn. (3.7)
Ec = Zg +
v2/2
Ec
= 35 x 9.81 + 62/2
= 343.4 + 18
= 361.4 J
Therefore total power
required
=
Ec x mass rate of flow
= EcVr
= 361.4 x 2.7 x 10-2 x 1000 J s-1
= 9758 J s-1
and, since
1 h.p. = 7.46 x 102 J s-1,
required power
= 13 h.p.
 Fluid-flow
theory > VISCOSITY
 Back
to the top |
Mass
balance 
