The
analysis of filtration is largely a question of studying the flow system.
The fluid passes through the filter medium, which offers resistance to
its passage, under the influence of a force which is the pressure differential
across the filter. Thus, we can write the familiar equation:
rate of filtration = driving force/resistance
Resistance
arises from the filter cloth, mesh, or bed, and to this is added the resistance
of the filter cake as it accumulates. The filter-cake resistance
is obtained by multiplying the specific resistance of the filter cake,
that is its resistance per unit thickness, by the thickness of the cake.
The resistances of the filter material and pre-coat are combined into
a single resistance called the filter resistance. It is convenient to
express the filter resistance in terms of a fictitious thickness of filter
cake. This thickness is multiplied by the specific resistance of the filter
cake to give the filter resistance. Thus the overall equation giving the
volumetric rate of flow dV/dt is:
dV/dt = (ADP)/R
As the total resistance
is proportional to the viscosity of the fluid, we can write:
R = mr(Lc
+ L)
where
R is the resistance to flow through the filter, m
is the viscosity of the fluid, r is the specific resistance of
the filter cake, Lc is the thickness of the filter cake
and L is the fictitious equivalent thickness of the filter cloth
and pre-coat, A is the filter area, and DP
is the pressure drop across the filter.
If
the rate of flow of the liquid and its solid content are known and assuming
that all solids are retained on the filter, the thickness of the filter
cake can be expressed by:
Lc = wV/A
where
w is the fractional solid content per unit volume of liquid, V
is the volume of fluid that has passed through the filter and A
is the area of filter surface on which the cake forms.
The resistance can
then be written
R = mr[w(V/A)
+ L)
(10.11)
and the equation
for flow through the filter, under the driving force of the pressure drop
is then:
dV/dt = ADP/mr[w(V/A)
+ L]
(10.12)
Equation
(10.12) may be regarded as the fundamental equation for filtration. It
expresses the rate of filtration in terms of quantities that can be measured,
found from tables, or in some cases estimated. It can be used to predict
the performance of large-scale filters on the basis of laboratory or pilot
scale tests. Two applications of eqn. (10.12) are filtration at a constant
flow rate and filtration under constant pressure.
Constant-rate Filtration
In
the early stages of a filtration cycle, it frequently happens that the
filter resistance is large relative to the resistance of the filter cake
because the cake is thin. Under these circumstances, the resistance offered
to the flow is virtually constant and so filtration proceeds at a more
or less constant rate. Equation (10.12) can then be integrated to give
the quantity of liquid passed through the filter in a given time. The
terms on the right-hand side of eqn.(10.12) are constant so that integration
is very simple:
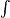 dV/Adt
= V/At = DP/mr[w(V/A)
+ L]
or DP
= V/At x mr[w(V/A)
+ L]
(10.13)
From
eqn. (10.13) the pressure drop required for any desired flow rate can
be found. Also, if a series of runs is carried out under different pressures,
the results can be used to determine the resistance of the filter cake.
Constant-pressure Filtration
Once
the initial cake has been built up, and this is true of the greater part
of many practical filtration operations, flow occurs under a constant-pressure
differential. Under these conditions, the term DP
in eqn. (10.12) is constant and so
mr[w(V/A)
+ L]dV = ADPdt
and integration from
V = 0 at t = 0, to V = V at t = t
mr[w(V2/2A)
+ LV] = ADPt
and rewriting this
tA/V = [mrw/2DP]
x (V/A) + mrL/DP
t
/ (V/A) = [mrw/2DP]
x (V/A) + mrL/DP
(10.14)
Equation
(10.14) is useful because it covers a situation that is frequently found
in a practical filtration plant. It can be used to predict the performance
of filtration plant on the basis of experimental results. If a test is
carried out using constant pressure, collecting and measuring the filtrate
at measured time intervals, a filtration graph can be plotted of
t/(V/A) against (V/A) and from the statement of eqn.
(10.14) it can be seen that this graph should be a straight line. The
slope of this line will correspond to mrw/2DP
and the intercept on the t/(V/A) axis will give the value
of mrL/DP.
Since, in general, m,
w, DP
and A are known or can be measured, the values of the slope and
intercept on this graph enable L and r to be calculated.
 EXAMPLE
10.6. Volume of filtrate from a filter press
A filtration test was carried out, with a particular product slurry, on
a laboratory filter press under a constant pressure of 340 kPa and volumes
of filtrate were collected as follows:
| Filtrate
volume (kg) |
20
|
40
|
60
|
80
|
| Time
(min) |
8
|
26
|
54.5
|
93
|
The
area of the laboratory filter was 0.186 m2. In a plant scale
filter, it is desired to filter a slurry containing the same material,
but at 50% greater concentration than that used for the test, and under
a pressure of 270 kPa. Estimate the quantity of filtrate that would pass
through in 1 hour if the area of the filter is 9.3 m2.
From the experimental data:
| V
(kg) |
20
|
40
|
60
|
80
|
| t
(s) |
480
|
1560
|
3270
|
5580
|
| V/A
(kg/m2) |
107.5
|
215
|
323
|
430
|
| t/(V/A)
(s m2 kg-1) |
4.47
|
7.26
|
10.12
|
12.98
|
These values of t/(V/A) are plotted against the corresponding values
of V/A in Fig. 10.7. From the graph, we find that
the slope of the line is 0.0265, and the intercept 1.6.
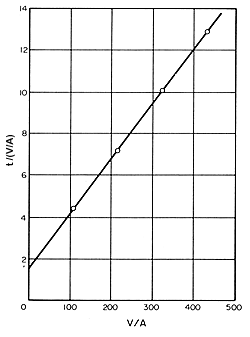
FIG. 10.7 Filtration Graph
Then substituting in eqn. (10.14) we have
t/(V/A)
= 0.0265(V/A) + 1.6.
To
fit the desired conditions for the plant filter, the constants in this
equation will have to be modified. If all of the factors in eqn. (10.14)
except those which are varied in the problem are combined into constants,
K and K', we can write
t/(V/A) = (w/DP)Kx(V/A)
+ K'/DP
(a)
In the laboratory
experiment w = w1, and DP
= DP1
K = (0.0265DP1
/w1) and K' = 1.6DP1
For
the new plant condition, w = w2 and P
= P2, so that, substituting in the eqn.(a) above, we
then have for the plant filter, under the given conditions:
t/(V/A)
= (0.0265 DP1/w1)(w2/DP2)(V/A)
+ (1.6DP1)(1/DP2)
and since from these
conditions
DP1/DP2
= 340/270
and w2/w1
= 150/100,
t/(V/A)
= 0.0265(340/270)(150/100)(V/A) + 1.6(340/270)
= 0.05(V/A) + 2.0
t = 0.5(V/A)2 + 2.0(V/A).
To find the volume
that passes the filter in 1 h which is 3600 s, that is to find V for t
= 3600.
3600 = 0.05(V/A)2
+ 2.0(V/A)
and solving this
quadratic equation, we find that V/A = 250 kg m-2
and so the slurry
passing through 9.3 m2 in 1 h would be:
= 250 x 9.3
= 2325 kg.
Filter-cake Compressibility
With
some filter cakes, the specific resistance varies with the pressure drop
across it. This is because the cake becomes denser under the higher pressure
and so provides fewer and smaller passages for flow. The effect is spoken
of as the compressibility of the cake. Soft and flocculent materials provide
highly compressible filter cakes, whereas hard granular materials, such
as sugar and salt crystals, are little affected by pressure. To allow
for cake compressibility the empirical relationship has been proposed:
r = r'DPs
where
r is the specific resistance of the cake under pressure P,
DP
is the pressure drop across the filter, r' is the specific resistance
of the cake under a pressure drop of 1 atm and s is a constant
for the material, called its compressibility.
This
expression for r can be inserted into the filtration equations,
such as eqn. (10.14), and values for r' and s can be determined
by carrying out experimental runs under various pressures.
Filtration Equipment
The basic requirements
for filtration equipment are:
 mechanical
support for the filter medium,
flow
accesses to and from the filter medium and
provision
for removing excess filter cake.
In
some instances, washing of the filter cake to remove traces of the solution
may be necessary. Pressure can be provided on the upstream side of the
filter, or a vacuum can be drawn downstream, or both can be used to drive
the wash fluid through.
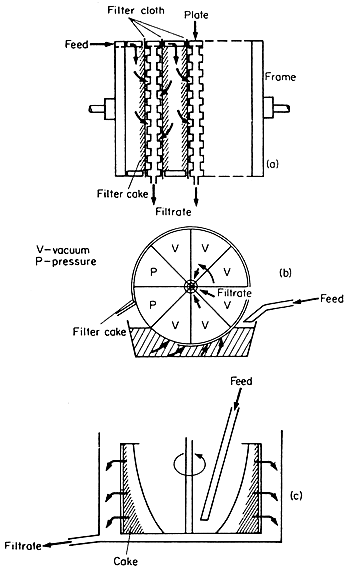
FIG. 10.8 Filtration equipment: (a) plate and frame press (b) rotary
vacuum filter (c) centrifugal filter
Plate and frame filter press
In
the plate and frame filter press, a cloth or mesh is spread out over plates
which support the cloth along ridges but at the same time leave a free
area, as large as possible, below the cloth for flow of the filtrate.
This is illustrated in Fig. 10.8(a). The plates with
their filter cloths may be horizontal, but they are more usually hung
vertically with a number of plates operated in parallel to give sufficient
area.
Filter
cake builds up on the upstream side of the cloth, that is the side away
from the plate. In the early stages of the filtration cycle, the pressure
drop across the cloth is small and filtration proceeds at more or less
a constant rate. As the cake increases, the process becomes more and more
a constant-pressure one and this is the case throughout most of the cycle.
When the available space between successive frames is filled with cake,
the press has to be dismantled and the cake scraped off and cleaned, after
which a further cycle can be initiated.
The
plate and frame filter press is cheap but it is difficult to mechanize
to any great extent. Variants of the plate and frame press have been developed
which allow easier discharging of the filter cake. For example, the plates,
which may be rectangular or circular, are supported on a central hollow
shaft for the filtrate and the whole assembly enclosed in a pressure tank
containing the slurry. Filtration can be done under pressure or vacuum.
The advantage of vacuum filtration is that the pressure drop can be maintained
whilst the cake is still under atmospheric pressure and so can be removed
easily. The disadvantages are the greater costs of maintaining a given
pressure drop by applying a vacuum and the limitation on the vacuum to
about 80 kPa maximum. In pressure filtration, the pressure driving force
is limited only by the economics of attaining the pressure and by the
mechanical strength of the equipment.
Rotary filters
In
rotary filters, the flow passes through a rotating cylindrical cloth from
which the filter cake can be continuously scraped. Either pressure or
vacuum can provide the driving force, but a particularly useful form is
the rotary vacuum filter. In this, the cloth is supported on the periphery
of a horizontal cylindrical drum that dips into a bath of the slurry.
Vacuum is drawn in those segments of the drum surface on which the cake
is building up. A suitable bearing applies the vacuum at the stage where
the actual filtration commences and breaks the vacuum at the stage where
the cake is being scraped off after filtration. Filtrate is removed through
trunnion bearings. Rotary vacuum filters are expensive, but they do provide
a considerable degree of mechanization and convenience. A rotary vacuum
filter is illustrated diagrammatically in Fig. 10.8(b).
Centrifugal filters
Centrifugal
force is used to provide the driving force in some filters. These machines
are really centrifuges fitted with a perforated bowl that may also have
filter cloth on it. Liquid is fed into the interior of the bowl and under
the centrifugal forces, it passes out through the filter material. This
is illustrated in Fig. 10.8(c).
Air filters
Filters
are used quite extensively to remove suspended dust or particles from
air streams. The air or gas moves through a fabric and the dust is left
behind. These filters are particularly useful for the removal of fine
particles. One type of bag filter consists of a number of vertical cylindrical
cloth bags 15-30 cm in diameter, the air passing through the bags in parallel.
Air bearing the dust enters the bags, usually at the bottom and the air
passes out through the cloth. A familiar example of a bag filter for dust
is to be found in the domestic vacuum cleaner. Some designs of bag filters
provide for the mechanical removal of the accumulated dust. For removal
of particles less than 5 mm
diameter in modern air sterilization units, paper filters and packed tubular
filters are used. These cover the range of sizes of bacterial cells and
spores.
 Mechanical
separations > SIEVING
 Back
to the top |
Plate and frame filter press 